|

According to
Special relativity, time no longer is an absolute quantity. It means
we cannot say if an event is simultaneous for everyone. It is
simultaneous for people in the same
reference frame but not simultaneous for people with different reference point.
Sounds
confusing??
O.k , First
of all, let us see what simultaneous events are. We can say that two events are
simultaneous if they occur exactly at the same time. If the events occur very
close to each other, we can easily distinguish whether that event is
simultaneous or not. But if they occur at far away from each other, how can we
say that they are simultaneous?
For that we
must take into account what time they take place.
Einstein did some "thought" experiment to illustrate the important
principles. Suppose there is an observer called O, situated at point O, the
midpoint of AB (see figure 2-1). At A and B, a light bulb is turned on at each
end. Suppose they were turned on at the same time. Observer O will see the light
when the light reaches him. Since light travels at constant speed and the
distance OB equals OA, the time it takes for the light to travel from A to O and
from B to O is the same. Therefore, Observer O can conclude that the two light
bulbs were turned on at the same time.

Now consider two different Observers, O1 and O2, moving
with a velocity with v with respect to each other. They are on a train traveling
in opposite direction, so that to O2, O1 is
moving the right with velocity v. On the other hand, O2 is moving to
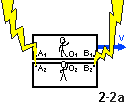
the left with velocity v. Now lightning strikes on both trains at A1
and B1 on O1's train and A2 and B2
on O2's train (see diagram on the left). O1 and O2
are happened to be midway between A1B1 and A2B2
respectively. Before we go on, let us put ourselves in O2's reference
frame and assume that the two events occurred simultaneously in this reference
frame at the time when O1 and O2 are opposite each other,
as shown in figure 2-2a. After a short time, the light reaches O2 at
the same time, as shown in figure 2-2b. Since O2 is the midpoint of A2B2,
he knows that the two events occurred simultaneously in his reference frame.
When light travels to O2, O1 is moving to the right. Then
by the time the light reaches O2, the light from B1 has
already passed O1 and that from B2 has not yet reached O1.
Hence, O1 claims that the lightning strikes at B1 before
it does at B2. From the result, we conclude that the two events that
are simultaneous to one observer are not simultaneous to the other observer in a
different reference frame. Time and simultaneity are relative.
We
are hardly aware of the relative nature of simultaneity. It only becomes
noticeable when the distance between the event and the observer is huge or when
the relative velocity of the two reference frame is close to speed of light.
|